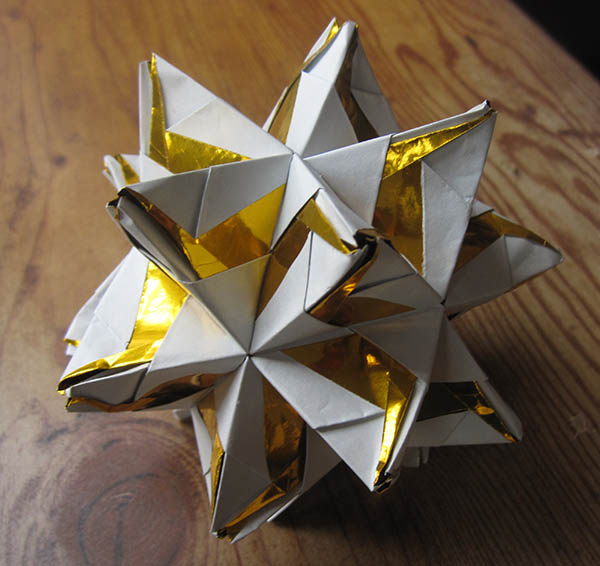
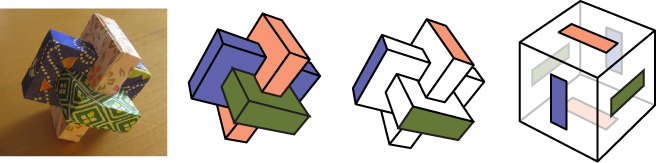
This is an appendix to my series about symmetry in origami. Here I will provide a proof that my construction of symmetrical colorings works.
While I try to make the series accessible to people who do not know much about math, I don’t think there’s much point in trying to make this proof broadly accessible. This is intended as a reference for people with some experience with group theory. (You need to know about cosets at the very least.)