This is the second part of a series about symmetry in origami. Here I talk about the role colors play in reducing symmetry.
Let’s return to the ninja star that I showed you last time. I said that it has a symmetry group of order 4, because there are four transformations preserve the shape of the ninja star: rotation by 0, 90, 180, or 270 degrees.
But suppose we want to preserve more than the ninja star’s shape. We also want to preserve its color. The only tranformations that preserve shape and color are rotations by 0 and 180 degrees. So the ninja star actually has two kinds of symmetry groups: the shape symmetry group of order 4, and the color symmetry group of order 2.
The color symmetry group is always a subset of the shape symmetry group. We have a special name for groups which are subsets of other groups, we call them subgroups.

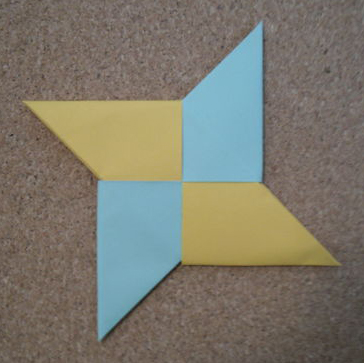


 Fig. 1: Cat of ambiguous state. Credit:
Fig. 1: Cat of ambiguous state. Credit: