This is the second part of a series about symmetry in origami. Here I talk about the role colors play in reducing symmetry.
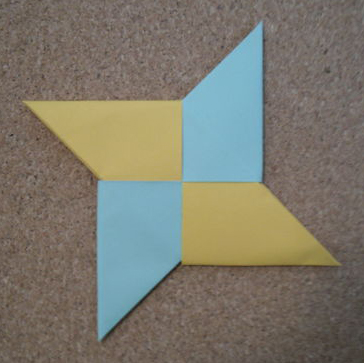
Let’s return to the ninja star that I showed you last time. I said that it has a symmetry group of order 4, because there are four transformations preserve the shape of the ninja star: rotation by 0, 90, 180, or 270 degrees.
But suppose we want to preserve more than the ninja star’s shape. We also want to preserve its color. The only tranformations that preserve shape and color are rotations by 0 and 180 degrees. So the ninja star actually has two kinds of symmetry groups: the shape symmetry group of order 4, and the color symmetry group of order 2.
The color symmetry group is always a subset of the shape symmetry group. We have a special name for groups which are subsets of other groups, we call them subgroups.
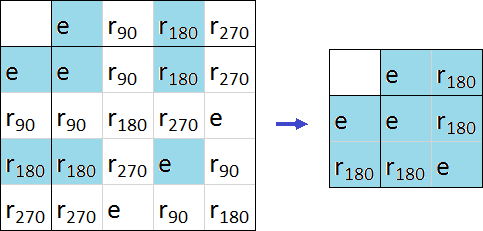
On the left is the multiplication table for the shape symmetry group; on the right is the one for the color symmetry group. Note that the one on the right takes a subset of the rows and columns from the table on the left.
Some facts about subgroups:
-
- Every group is a subgroup of itself. Suppose the ninja star were all one color, then the shape symmetry group is identical to the color symmetry group. And therefore, they are subgroups of each other.
- The trivial group is the group containing nothing but the identity transformation (i.e. rotation by 0 degrees). The trivial group is a subgroup of every group. So we could paint the ninja star a bunch of random colors, and then the color symmetry group would be the trivial group.
- If you take the order of a group, then it is always divisible by the order of its subgroups. This is known as Lagrange’s theorem. For example, the ninja star has a shape symmetry group of order 4. Therefore the color symmetry group must have order 1, 2, or 4.
Now let’s talk about a few other examples.
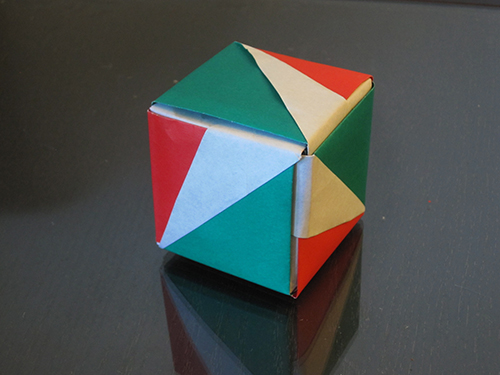
Ray Cube by Meenakshi Mukerji. Three faces are showing, but there are also three faces in the back which you can’t see. The faces in the back are a completely different color. Let’s say they’re purple.
This Ray Cube has cubic symmetry. If we include only rotations, then the symmetry group has order 24. But if we also include reflections, then the symmetry group has order 48. Now what about the color symmetry group? There’s a set of 3 rotations, all around the same axis, which will lead to the preservation of the cube’s color. The rotation axis is shown below. You may rotate around this axis by 0, 120, or 240 degrees.
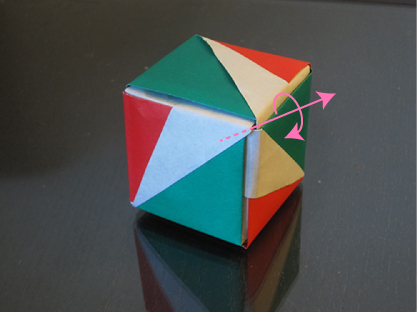
This is the rotation axis of the cube’s color symmetry.
So the shape symmetry group has order 48, while the color symmetry group has order 3. Sanity check: is 48 divisible by 3? Yes it is! Math works!
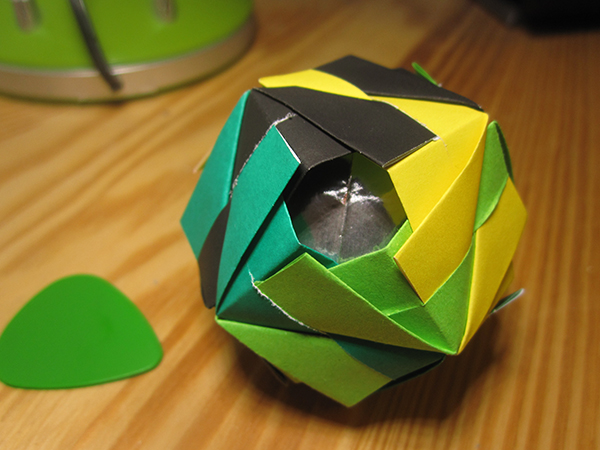
Etna Kusudama by Ekaterina Lukasheva. The original design had icosahedral symmetry, but my version has cubic symmetry.
Here’s another example, my Etna Kusudama. Note that if you rotate the model by 90 degrees, all the colors change, yellow to black, black to dark green, dark green to light green, and light green to yellow. But there’s something that doesn’t change, which is the pattern that the colors make. I declare this to be yet another symmetry group, the pattern symmetry group. The color symmetry group is a subgroup of the pattern symmetry group, which is a subgroup of the shape symmetry group. Or, in mathematical notation:
color symmetry pattern symmetry
shape symmetry
It’s difficult to determine the symmetry groups of the Etna Kusudama because the photo only shows one side. So here’s a skeletal diagram of the coloring:
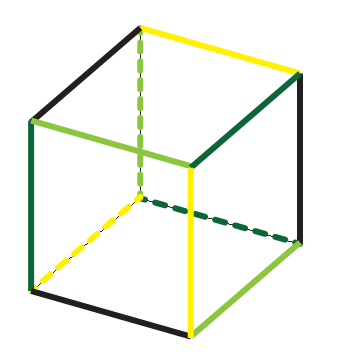
A skeletal diagram showing how to use 4 colors to symmetrically color the edges of a cube.
One thing that is considered aesthetically desirable in origami is a “symmetric” coloring. There isn’t a clear way to define a symmetric coloring, but I propose the following criterion: The pattern symmetry group must be large, and the color symmetry group must be small.
For the Etna Kusudama, the shape symmetry group is the full cubic symmetry group, order 48. The pattern symmetry group is the cubic symmetry group without reflections, order 24. The color symmetry group is the trivial group of order 1. This sounds like a symmetric coloring to me!
An interesting math problem would be to determine the possible symmetric colorings of regular polyhedra. And by “interesting” I mean that literally nobody knows the answer, as far as I know. However, I did once see a blogger consider the question using slightly different definitions, so the answer is probably within reach.

Well, please do share it, if you come across anything like that. I enjoy it for its own sake, but there are some nice ways to apply group theory and so forth to music as well. A lot of helpful results in topology are for 2D surfaces and such — while I know just a few extra dimensions is still relatively “low” in the grand scheme of things, getting a handle on it (especially as a non-mathematician) is definitely a challenge. Still lots of unanswered questions about so many deceptively “simple” problems.
It would be interesting to work out what effect different folding procedures have — whether it’s equivalent when (hypothetically) turned inside out, questions like that. I don’t know much about origami, although I’d assume obscured features would often be symmetrical just as the exterior is; but it seems more difficult to understand how that would affect the relevant groups/subgroups. For instance, the triangles on the cube surface aren’t congruent, and presumably each type of paper fits into the pattern differently than the others, since it plays a different structural role or whatever — it’s no great mystery if you put it together, but for all I know the remaining paper could be doing something rather different/unexpected on the inside. Also…. if each piece of paper can have two different colors, one on each side, then you could consider how different combinations work together.
@Consciousness Razor
Yeah, I was thinking I would do that. There are only so many finite spherical symmetry groups, so in principle this is a O(1) problem.
It depends on the model, but usually the symmetry of the internal structure is the same as the symmetry of the externally visible structure. Well, sometimes I use chiral units to build a structure that is externally achiral. The Etna Kusudama is an example. I think you can actually see in the photo that the coloring patterns are inherently chiral, which has to do with the internal structure.
I was thinking that in addition to shape symmetry, pattern symmetry, and color symmetry, I could identify a fourth symmetry group which I’ll call structural symmetry. Unfortunately this is hard to illustrate since by its very nature, it is hidden.