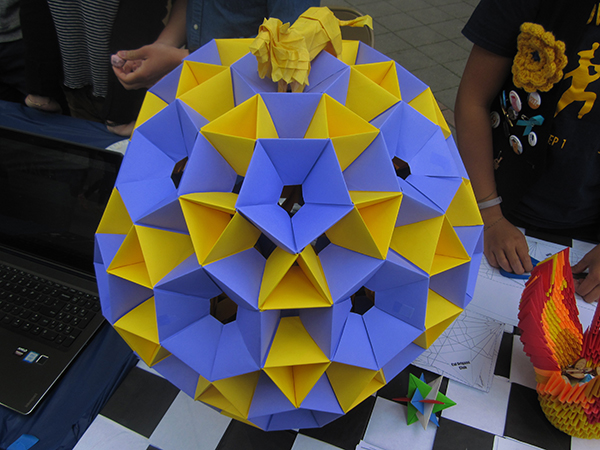
Double Sided Concave Hexagonal Ring Solid by Tomoko Fuse
The local origami club wanted to make something big for an activities fair, so we worked together to fold 360 pieces and assemble them together. You can see the result above (along with a few other models they had for display). Assembly was quite tricky, because at this size the weight of the model pulls itself apart.
I don’t think this polyhedron has a name. Let’s see, there are 20 hexagons, 12 pentagons, 90 squares, and 60 triangles. All in all, there are 182 faces, 360 edges, and 180 vertices.
One thing I find interesting about this polyhedron, is that it’s technically concave (i.e. some edges bend inwards). You can see this by carefully looking at some of the vertices. Square + Triangle + Square + Hexagon, that’s 90 + 60 + 90 + 120 = 360 degrees. That means that these vertices could be flattened out. Of course, the vertices are not flat, and the only possible way for them to be non-flat is if some edges curve inward.

It’s a model of the universe!
Unless I’m mistaken, it’s related to a truncated icosahedron (AKA, soccer ball), which has 20 hexagons, 12 pentagons and … wait for it!…. 90 edges and 60 vertices.
So, you essentially replace each edge with a square and each vertex with a triangle. Sounds maybe a little crazy and hard to picture (without the origami picture above of course), but I think that would do it. I sort of doubt it has a proper name — probably a dozen syllables tossed together like a Greek salad if there is one.
In a sense, this already happens when you try to physically make the skeleton of a truncated icosahedron, which needs to have some nonzero width if it’ll be made of anything. That’s where the vertices and edges would be, but you can’t really make them 0- or 1-dimensional, although you can sort of get away with imagining the structure as very thin/small 2D surfaces (but even that’s not literally true)…. Anyway, in the ordinary case the skeleton is made of rectangles (joined by equilateral triangles) instead of squares. So you just make them squares. Doing that, with all of the polygons scaled as they are, is I suppose what introduces the concave weirdness.
@consciousness razor,
If you look at the Archimedean solids, there are a few that replace edges and vertices of a smaller polyhedron with polygons. It seems the naming convention is to add the prefix “small rhomb-” or “great rhomb-“. So if I were to give this solid a silly name, it would be “small rhombtruncated icosahedron”.
That’s a perfectly cromulent name, if you ask me. It’s not convex (not in this form), so I think some would insist on additional silliness.
The symmetry group is fairly interesting, because some vertices (1/3 of them) are at one of the pentagons while the rest are at a hexagon. So I guess that qualifies as “2-uniform,” because they are all regular polygons but vertices come in two different classes.
[Siggy]:
I’ve also been wrestling with a proper name for this shape.
If we compare the [Dodecahedron] to the [Rhombi-Cosi-Dodecahedron], we can see that the same trick has been done here
(IE: Edges become Squares & Corners become Triangles)
… to the [Truncated Icosahedron], but calling this a [“Rhombi-Cosi”-ed Truncation of an Icosahedron] just feels too awkward to me.
[Siggy]:
What strikes me is that all 60 Triangles plus 60Squares plus all 12 Pentagons can combine to make 12 Decagons.
This simplifies the structure into: 30 Squares, 20 Hexagons, & 12 Decagons.
[UPDATE-2019/04/28Su]:

A posting on my “FB”-page got a reply from [Matt Giallourakis], who was able to identify this shape as the
[Expanded Truncated Icosahedron].
Mystery Solved — Huzzah!
[Siggy]:
#IfYouDon’tMindMyAsking
Is there a video, or a website, that instructs us on how to make the module that you used, to make this model?
@DMC_Run #8,
You can search “Double Sided Concave Hexagonal Ring Solid”–which is the name of the module, not the polyhedron. Here’s one video.