Rat from the comic strip Pearls Before Swine can be forgiven for thinking that there is something supernatural going on. Everyone has experienced this. You carefully put away items that have long strings and cords and the next time you take them out, even after a brief interval, the cords are all entangled with each other and knotted. It seems almost like they have lives of their own, going wandering as soon as they are out of your sight. The blog Seriously, Science? points to a paper by two physicists that looked into this annoying and perplexing issue.
Here’s some text from the abstract of the paper.
It is well known that a jostled string tends to become knotted; yet the factors governing the “spontaneous” formation of various knots are unclear. We performed experiments in which a string was tumbled inside a box and found that complex knots often form within seconds. [My italics-MS] We used mathematical knot theory to analyze the knots. Above a critical string length, the probability P of knotting at first increased sharply with length but then saturated below 100% … The relative probability of forming a knot decreased exponentially with minimum crossing number and Mobius energy, mathematical measures of knot complexity. Based on the observation that long, stiff strings tend to form a coiled structure when confined, we propose a simple model to describe the knot formation based on random “braid moves” of the string end. Our model can qualitatively account for the observed distribution of knots and dependence on agitation time and string length.
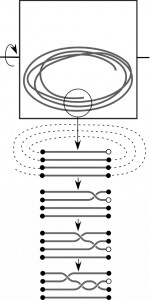
This figure and its caption from the paper gives the basic idea.
[Caption: Schematic illustration of the simplified model for knot formation. Because of its stiffness, the string tends to coil in the box, as seen in Fig. 1, causing a number of parallel string segments to lie parallel adjacent the end segment. As discussed in the text, we model knots as forming due to a random series of braid moves of the end segment among the adjacent segments (diagrams at bottom). The overall connectivity of the segments is indicated by the dashed line.]
That’s science, baby! Answering the important questions that puzzle everyone.

Haha, well for me this is fascinating because I work with ropes and so on in my daily life on a farm. I’m also a braider… I even can tie some of those knots that mathematical knot theory talks about.
I learned a long time ago how to coil up my ropes and so on to prevent tangling and nothing annoys me more than someone trying to “help” me by coiling them for me. It can take up a lot of time trying to get them lined out again. Everybody thinks I am ridiculously picky but it really saves time if a hose is coiled up so that it won’t tangle as you drag it out.
What about strings of Christmas lights?
What with the bulbs and plugs…
There are mathematicians that specialize in figuring out how to tell real knots from mere (?I don’t know what it’s called?) -- a real knot being something that locks solid instead of pulling apart. I believe it’s a part of the discipline of topology -- the field whose proponents allegedly don’t see much difference between a coffee cup and a donut.
The question of headphone wires comes down to making connectors that make it easy to disconnect and unspool the wire. The problem is that good connectors are expensive compared to wires. My old Grado Labs headphones do not have problems with the wires, neither do old David Clark pilot headsets -- why? You use a slightly more expensive, heavier wire with a heavier housing and the wire is less mobile and it’s not a problem at all. That’s one of the reasons I find it slightly ironic that Apple is trying to get the world to buy hundreds of millions of dollars more cheap headphones in order to solve the problem of: cheap headphones.
Marcus,
Yes, it is the field of topology and much of it deals with the connectedness of the points that make up the shape. The reason that a doughnut and a coffee cup are topologically equivalent is that you can take a doughnut and deform it to get something in the shape of a cup (and vice versa) without any cutting and/or pasting. The key point is that both the doughnut and the cup have one hole each. A doughnut is not topologically equivalent to a saucer because you cannot deform one into the other. You would have to make a hole in the saucer to be able get something in the shape of a doughnut or a coffee cup.
The problem is all in the twist/torque of the linear structure—string, rope, wire, hose—being coiled. Ensure that the structure has no twist out of line with the direction of the coil and you’ll never have a tangle.
This is one of the first lesson I learned sailing and in the Navy. kinked/twisted/fouled lines can get you killed.
Marcus, the word you are looking for is *unknot* (yes, mathematicians can play at the stupid-technical-names game as well).
Somewhere when sailing, I learned that tangled lines (sheets) can be avoided by feeding them into a buckle without attempting to coil them. This is handy when they needed to be free running. Coiling introduces a torque that will lead to kinking. If hoses are laid into a figure-eight patterns, they can be played out by holding the end and walking away.
I always put it down to being because there are so many more ways for a cable to be tangled than not tangled, so left to jumble unconstrained it is much more likely to occur than not.