You might be tempted to stare deeply into this image, trying to puzzle out what it is you’re looking at, but that’s how they get you.
That’s Haliphron, the 7-armed octopus, holding a jellyfish it’s been nibbling on. Now that I’ve told you, I hope that has broken the spell, and you’ll be able to escape. If not, well, a cephalopod has got to eat, and it’s next victim will by baffled by the way those twisting arms surround your face.

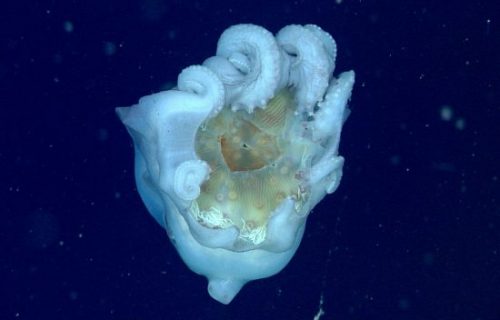

With seven tentacles, Haliphron can easily accommodate a six-dimensional Calabi-Yau shape, no doubt to confuse its prey.
Strictly speaking, since we live in a universe where space is curved (just not to the point where it is very noticeable on a day-to-day basis unless you’re in science-related academia) everything is non-Euclidean.
Speaking of axioms which lead to divergent mathematical systems, I remember reading a few years back that somebody had discovered logical consequences of the continuum hypothesis (that there is no set “bigger” than the integers but “smaller” than the reals) in regular, finite mathematics, which means that we could conceivably find a place where physics maps onto one of those finite cases and discover whether the continuum hypothesis is true or not in our particular universe, the same way we can detect the curvature of our universe’s geometry. Does anybody know if this has happened yet?
@ ^ The Vicar (via Freethoughtblogs) : ” ..everything is non-Euclidean.”
Except abstract mathematics notably the field of Euclidian geometry? / Pedantic nitnick mode.
There’s a place for that too alongside Lobachevskian geometry and Riemannian geometry, even if our Earth physically isn’t Flatland.